Simulation of a Lorentz (Thompson) Coilgun

Here are partial results of a simulation of a Lorentz coilgun, using FEMM for the magnetics and Matlab for the time simulations.

Simulation algorithm

Force on armature is determined with test currents at varying distances
Flux coupled through armature from driver coil is determined at varying distances
Inductance of driver coil is determined from integral(A.J) with test current
Inductance of armature (Cu ring) is determined, varying with position
Resistance of armature is determined
All of the above data is loaded into Matlab
Supply C and series R is chosen for approximately critically damped discharge of driver coil
Iterating over time:
- Coil current is simulated as a lumped LRC system; L varies (a little) with armature position
- Flux through the armature is calculated, change in flux gives armature EMF
- Armature current is simulated as a lumped LR system with a voltage source and L varying with position
- Force is determined from the two simulated currents and the current armature position
- Acceleration, velocity and position are found from force

The complete source (geometry, lua scripts, matlab source, simulation data) is here. It is released under GPL2.

Bad Assumptions

Steady-state magnetic simulation with no eddy currents. Test currents in magnetic sims were not very high, so iron may not have been as saturated (3T peak) as it will be in real life; these sims will be rerun.

Test Geometries

The simulations have been run with air core (no iron) and a long steel bar core passing through the driver coil and armature. Also planning on looking at more complex situations (fully shielded, tapering shields, ferromagnetic armatures, etc). Pictures when I get around to taking screenshots. In most of the simulations below, projectile mass is set at 6.1g (the mass of a Cu ring of the same size as in the FEMM simulations).

Results: Air Core

Probably I picked bad parameters, but the performance in this sim sucked. With relatively high coil inductance (27uH), you can see that the coil current pulse is too long (1.5ms) compared to the armature time constant (~0.3ms) and so the impulse is minimal: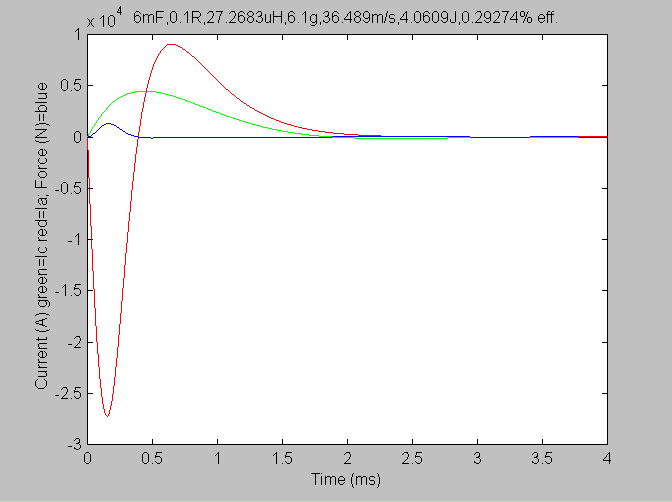

With a smaller inductance (6.8uH), the coil current correlates better with the armature current, but still not well. The main problem appears to be insufficient armature inductance; a heavier armature would reduce its Q and perhaps work a little better.

Results: Steel Core

With a steel core, flux coupling is much better though probably overestimated in the simulation due to the relatively small test currents used in FEMM. Very few turns are used and the driver pulses are much longer (nearly 2ms), but the increased inductance of the armature means that its time constant is much closer to that of the driver coil:

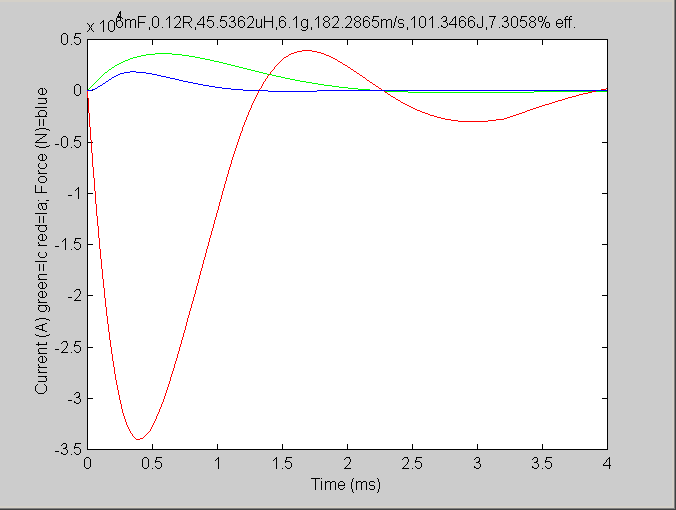
Better flux coupling gives similar peak armature currents, despite the slower coil current rise. Higher armature inductance and therefore longer current duration means that the repulsive force lasts much longer and the net impulse is much greater.

More coil turns, therefore more flux, higher EMF and higher armature Q, resulting in some suckback:

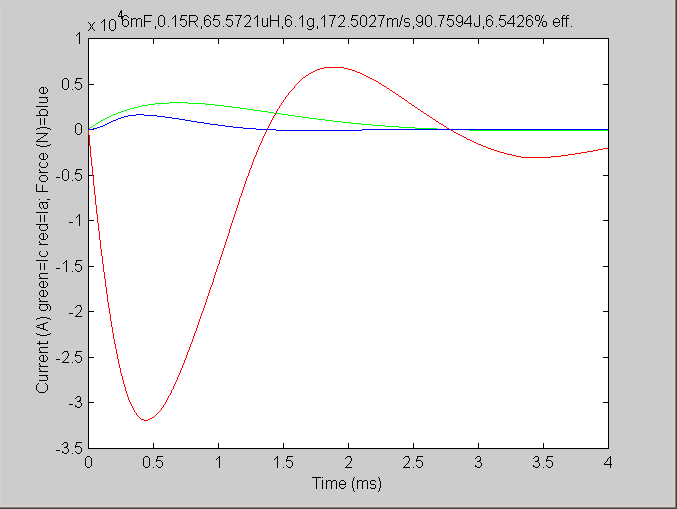
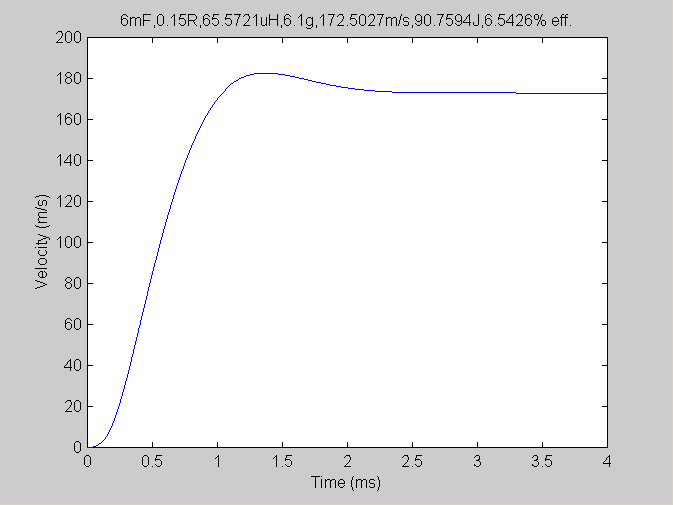
Fewer turns, armature a bit overdamped:

Observations

Back-EMF in the armature due to velocity has a significant effect on armature current - adjusting the speed via varying mass has significant effect on the armature current's Q. More mass => lower velocity => less back-EMF => lower Q. Lowering mass far enough can cause suckback due to dramatic armature current overshoot, even to the point where the armature will smack back into the coil at high speed.

Not Yet On This Webpage

Iterating results over varying armature mass, coil turns and source C. Results for other geometries: planar coils, more shielding, lower armature mass, increased armature diameter (including discs).